How do you calculate NPV?
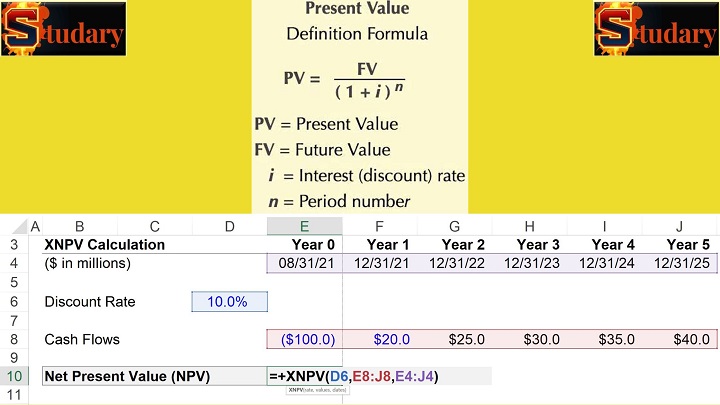
Net Present Value (NPV) is a financial metric that helps assess the profitability of an investment by comparing the present value of cash inflows to the present value of cash outflows. The formula for calculating NPV is:
NPV =
Σ [CF / (1 + r)^t]
- Initial Investment
Where:
CF = Cash Flow in year tr = Discount Ratet = Year
If the NPV is positive, the investment is potentially worthwhile as it generates more value than the initial cost. If it's negative, the investment might not be profitable enough.
How to calculate the IRR?
The Internal Rate of Return (IRR) is the discount rate at which the NPV of an investment becomes zero. It's the rate at which the present value of cash inflows equals the present value of cash outflows. The IRR is typically found through trial and error or by using financial software/tools.
- Definition: IRR is a financial metric used to determine the rate at which an investment's net present value (NPV) becomes zero.
- Concept: It represents the interest rate at which an investment breaks even, generating enough cash flows to cover its initial cost.
- Equation: IRR is found by solving for the discount rate that satisfies the equation where the sum of discounted cash inflows equals the initial investment.
- Trial and Error: IRR calculation often involves iterative approximation. You try different discount rates until NPV reaches approximately zero.
- Software/Tools: Financial calculators, spreadsheet software (like Excel), and specialized financial software can efficiently compute IRR.
- Complex Projects: In cases where cash flows change direction (negative to positive or vice versa), multiple IRRs might exist, making interpretation challenging.
- Decision Criterion: In investment decisions, if IRR is greater than the required rate of return (hurdle rate), the investment may be considered worthwhile.
- Relative Comparison: When evaluating multiple projects, comparing IRRs can help choose the one with the highest potential return.
- Limitations: IRR assumes reinvestment of cash flows at the computed rate, which might not be practical or accurate.
- Negative IRR: Negative IRR indicates that the project's costs exceed potential returns; such projects should generally be avoided.
- NPV vs. IRR: While NPV gives an absolute value of investment profitability, IRR offers a percentage return. In cases of conflicting decisions, NPV is a more reliable indicator.
What is NPV and IRR examples?
Example: You're considering an investment that requires an initial investment of $10,000. Over the next three years, you expect cash inflows of $4,000, $4,500, and $5,000. The discount rate is 10%. Calculate NPV and IRR.
NPV = (4000 / (1 + 0.10)^1) +
(4500 / (1 + 0.10)^2) + (5000 /
(1 + 0.10)^3) - 10000
NPV ≈ $2,727.57
- IRR: You would need to use trial and error or software to find the IRR, which in this case is approximately 19.72%.
How do you calculate IRR from NPV in Excel?
In Excel, you can use the
IRR function to directly calculate the Internal Rate of Return from a series of cash flows. For example, if your cash flows are in cells
A1 through
A5, you can use the formula:
How to calculate NPV and IRR in Excel:
- List your cash flows in a column.
- Use the
NPV function to calculate NPV, for example:
=NPV(discount_rate,
range_of_cash_flows)
- Use the
IRR function to calculate IRR, for example:
=IRR(range_of_cash_flows)
How to calculate NPV and IRR with examples:
Refer to the earlier provided investment example for a step-by-step calculation of NPV and IRR.
Investment Example
|
|
| Initial Investment |
$10,000 |
| Cash Flows (Year) |
Year 1 |
| Cash Inflows |
$4,000 |
| Discount Rate |
10% |
Calculation
|
|
| Year 1 |
Year 2 |
| Cash Flow |
$4,000 |
| Present Value Factor (1 + r)^t |
(1 + 0.10)^1 = 0.9091 |
| Discounted Cash Flow |
$4,000 * 0.9091 = $3,636.40 |
NPV Calculation
|
|
| NPV = Sum of Discounted Cash Flows - Initial Investment |
|
| NPV = ($3,636.40 + $3,721.80 + $3,756.50) - $10,000 |
|
| NPV ≈ $2,727.70 |
|
IRR Calculation
|
|
| Finding the rate that makes NPV zero |
|
| Try different rates (trial and error) |
|
| IRR ≈ 19.72% |
|
This table outlines the step-by-step calculations of NPV and IRR for the given investment example.
Relationship between NPV and IRR:
NPV and IRR both assess the profitability of an investment, but they can sometimes lead to conflicting results. NPV assumes a specific discount rate, while IRR finds the discount rate that makes NPV zero. In cases of mutually exclusive projects, NPV is generally a more reliable metric.
- Profitability Assessment: Both NPV (Net Present Value) and IRR (Internal Rate of Return) evaluate the profitability of investment projects.
- Conflict Potential: NPV and IRR can occasionally provide conflicting recommendations about whether to accept an investment.
- NPV Assumption: NPV uses a predetermined discount rate (often a company's cost of capital) to calculate the present value of cash flows. It directly measures the value added by an investment.
- IRR Goal: IRR aims to find the discount rate at which the investment's NPV becomes zero. It represents the project's internal rate of growth.
- Multiple IRRs: Some projects have irregular cash flows that result in multiple IRRs, making IRR interpretation complex.
- Mutually Exclusive Projects: When choosing between mutually exclusive projects, NPV is usually the preferred criterion. It provides a clear indication of which project adds more value in absolute terms.
- Reinvestment Assumption: IRR assumes that positive cash flows are reinvested at the calculated rate, which might not be realistic. NPV doesn't make this assumption.
- Size and Scale: NPV considers the absolute dollar value of the investment, making it more useful for comparing projects of different sizes.
- Decision Rule for IRR: If IRR is greater than the required rate of return, the project might be accepted; if lower, it might be rejected.
- Decision Rule for NPV: If NPV is positive, the project is generally considered acceptable, as it adds value. If negative, it may not be a good investment.
- Consistency with Goals: If the goal is to maximize value, NPV is often more reliable, as it considers absolute dollar gains.
- Changing Discount Rates: NPV adjusts for different discount rates for different projects, making it adaptable to changing market conditions.
- Overall Consideration: While both NPV and IRR have merits, NPV is favored for most investment decisions due to its logical consistency and alignment with value maximization goals.
Understanding the strengths and weaknesses of both NPV and IRR helps in making more informed investment decisions, especially when dealing with complex projects and competing opportunities.
Comparison Between NPV and IRR
| Aspect |
Description |
| Profitability Assessment |
Both assess investment returns |
| Conflict Potential |
Can sometimes conflict |
| NPV Assumption |
Uses a preset discount rate |
| IRR Goal |
Finds rate for NPV = 0 |
| Multiple IRRs |
Possible with complex cash flows |
| Mutually Exclusive Projects |
NPV preferred for clear choice |
| Reinvestment Assumption |
IRR assumes reinvestment |
| Size and Scale Consideration |
NPV suitable for varying sizes |
| Decision Rule for IRR |
IRR > Req. Rate = Potential Acceptance |
| Decision Rule for NPV |
Positive NPV = Generally Acceptable |
| Consistency with Value Maximization |
NPV aligns with maximizing value |
| Changing Discount Rates Consideration |
NPV adapts to market changes |
Understanding these points helps in making sound investment decisions based on the appropriate financial metric for the given situation.

How to calculate NPV and IRR using a financial calculator:
Most financial calculators have specific functions for NPV and IRR calculations. You would enter the cash flows, discount rate, and follow the calculator's instructions to get the results. Consult your calculator's manual for detailed guidance.
- Access Functions: Most financial calculators have dedicated NPV and IRR functions.
- Entering Cash Flows: Input the series of cash flows for each period (including the initial investment as a negative value).
- Entering Discount Rate: Input the discount rate (as a percentage or decimal) that corresponds to the cost of capital or required rate of return.
- Calculating NPV: Use the NPV function to compute the net present value based on the entered cash flows and discount rate.
- Calculating IRR: Use the IRR function to find the internal rate of return for the cash flows provided.
- Reading the Results: The calculator will display the computed NPV and IRR values.
- Interpreting Results: A positive NPV suggests the investment could be worthwhile. If IRR is greater than your required rate of return, the project might be acceptable.
- Consulting Manual: If unsure, consult your calculator's manual for specific instructions related to NPV and IRR calculations.
Using your financial calculator's functions can simplify complex calculations and provide accurate results for NPV and IRR evaluations.

